题意：
有一些圆形的镜子，一束光线射进来，试模拟光线的反射过程
测试数据：
/Files/yzhw/reflect.rar解法：
这个问题首先从整体上看应该用迭代法，每次确定v向量和初始位置，然后循环10次即可
下面解决2个问题：
1、求与某个圆的的第一个交点
2、求新的速度矢量
对以第一个问题，我们设参数k，然后列方程:
设x',y'为圆心坐标，r为半径。x0,y0为初始点，(dx,dy)为速度矢量
那么有
(x0+kdx-x')
2+(y0+kdy-y')
2=r
2
如果delta<0，那么说明直线与圆没有交点
否则取k=(-b-sqrt(delta))/2a
如果k<=0，则说明交点在射线的反方向或者交点就在原来的位置，不满足题意
第一个问题算比较好的解决了。
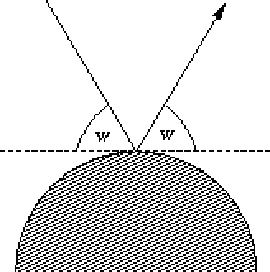
第二个问题我觉得比较简单的方法是利用向量的旋转矩阵
蓝色的向量和红色的向量在知道交点后是很容易算出的。cosθ利用向量夹角公式也不是问题，由于θ肯定是锐角，sinθ也不是问题了。
然后就可以利用旋转矩阵将红色的向量右旋得到目标速度向量（不一定是右旋，如果光线从红色向量右侧射入，那么就要左旋了。判断左右可以用叉积或者干脆直接将两个向量都求出，选择与原来那个不同的向量即可）。
旋转矩阵(左旋)：
[cosθ -sinθ]
[sinθ cosθ]
有一点要注意：精度卡到1e-8就够了，求sin的时候sqrt(1-cos*cos+
eps)，不然可能会出现-0.000000001这种情况。。。
代码：
1
# include <cstdio>
2# include <cmath>
3# include <cstdlib>
4using namespace std;
5# define eps 1e-8
6struct circle
7
{
8
double x,y,r;
9
}cir[30];
10int n,id[30];
11double tmp[30][4];
12double dx,dy,x0,y00;
13inline double dis(double x1,double y1,double x2,double y2)
14{
15 return (((x1)-(x2))*((x1)-(x2))+((y1)-(y2))*((y1)-(y2)));
16}
17inline bool less(double n1,double n2)
18{
19 return (fabs((n1)-(n2))>eps&&(n1)<(n2));
20}
21inline bool gt(double n1,double n2)
22{
23 return (fabs((n1)-(n2))>eps&&(n1)>(n2));
24}
25void cal(circle &pos,int &count,int _id)
26{
27 double b=2.0*((x0-pos.x)*dx+(y00-pos.y)*dy),a=dx*dx+dy*dy,c=(x0-pos.x)*(x0-pos.x)+(y00-pos.y)*(y00-pos.y)-pos.r*pos.r;
28 double delta=b*b-4.0*a*c;
29 if(less(delta,0)) return;
30 else
31
{
32 double k=(-b-sqrt(delta))/2.0/a;
33 if(!gt(k,0)) return;
34 id[count]=_id;
35 tmp[count][0]=x0+dx*k;
36 tmp[count][1]=y00+dy*k;
37 double x2=tmp[count][0]-pos.x,y2=tmp[count][1]-pos.y;//连心线向量
38 double x1=x0-tmp[count][0],y1=y00-tmp[count][1];//原向量
39 double cs=(x1*x2+y2*y1)/sqrt(x1*x1+y1*y1)/sqrt(x2*x2+y2*y2);//计算cos
40 double sn=sqrt(1-cs*cs);//计算sin
41 if(less(x1*y2-x2*y1,0))//点积判断左右情况
42 x1=cs*x2+sn*y2,y1=-sn*x2+cs*y2;
43 else if(gt(x1*y2-x2*y1,0))
44 x1=cs*x2-sn*y2,y1=sn*x2+cs*y2;
45 else x1=x2,y1=y2;
46 k=sqrt(x1*x1+y1*y1);
47 x1/=k;
48 y1/=k;
49 tmp[count][2]=x1;
50 tmp[count][3]=y1;
51 count++;
52
}
53}
54int main()
55{
56 //freopen("reflect.in","r",stdin);
57 //freopen("ans.txt","w",stdout);
58 int count=1;
59 while(true)
60 {
61 scanf("%d",&n);
62 if(!n) break;
63 for(int i=0;i<n;i++)
64 scanf("%lf%lf%lf",&cir[i].x,&cir[i].y,&cir[i].r);
65 scanf("%lf%lf%lf%lf",&x0,&y00,&dx,&dy);
66 printf("Scene %d\n",count++);
67 for(int t=1;t<=11;t++)
68 {
69 int c=0;
70 for(int i=0;i<n;i++)
71 cal(cir[i],c,i);
72 if(c==0)
73 {
74 printf("inf\n\n");
75 break;
76 }
77 else if(t!=11)
78 {
79 double len=1e16;
80 int target;
81 for(int i=0;i<c;i++)
82 {
83 if(less(dis(x0,y00,tmp[i][0],tmp[i][1]),len))
84 len=dis(x0,y00,tmp[i][0],tmp[i][1]),target=i;
85 }
86 printf("%d ",id[target]+1);
87 x0=tmp[target][0];
88 y00=tmp[target][1];
89 dx=tmp[target][2];
90 dy=tmp[target][3];
91 // printf("%.4f %.4f %.4f %.4f\n",x0,y00,dx,dy);
92 }
93 else printf("\n\n");
94 }
95 }
96 return 0;
97}
98
99
100