Taxi Cab Scheme
|
Time Limit: 1000MS
|
|
Memory Limit: 30000K
|
|
Total Submissions: 1342
|
|
Accepted: 587
|
Description
Running a taxi station is not all that simple. Apart from the obvious demand for a centralised coordination of the cabs in order to pick up the customers calling to get a cab as soon as possible,there is also a need to schedule all the taxi rides which have been booked in advance.Given a list of all booked taxi rides for the next day, you want to minimise the number of cabs needed to carry out all of the rides.
For the sake of simplicity, we model a city as a rectangular grid. An address in the city is denoted by two integers: the street and avenue number. The time needed to get from the address a, b to c, d by taxi is |a - c| + |b - d| minutes. A cab may carry out a booked ride if it is its first ride of the day, or if it can get to the source address of the new ride from its latest,at least one minute before the new ride's scheduled departure. Note that some rides may end after midnight.
Input
On the first line of the input is a single positive integer N, telling the number of test scenarios to follow. Each scenario begins with a line containing an integer M, 0 < M < 500, being the number of booked taxi rides. The following M lines contain the rides. Each ride is described by a departure time on the format hh:mm (ranging from 00:00 to 23:59), two integers a b that are the coordinates of the source address and two integers c d that are the coordinates of the destination address. All coordinates are at least 0 and strictly smaller than 200. The booked rides in each scenario are sorted in order of increasing departure time.
Output
For each scenario, output one line containing the minimum number of cabs required to carry out all the booked taxi rides.
Sample Input
2
2
08:00 10 11 9 16
08:07 9 16 10 11
2
08:00 10 11 9 16
08:06 9 16 10 11
Sample Output
1
2
Source
Northwestern Europe 2004
根据这道题目的意思,我们可以建一张图,对于两个booked taxi ride,ri和rj如果一辆车能够先完成ri的任务再有时间赶去完成rj的任务,那么就建立一条ri指向rj的边。
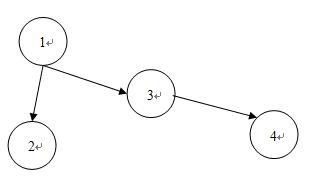
按照题目的要求,要选择最少的taxi来完成这些任务。显然在上面这个例子中,需要安排2辆taxi。结合这个图,可以把题目的要求转化为找出最少的路径条数,使得这些路径覆盖途中所有的边,例如可以选择2条路径1->3->4和1->2就可以覆盖所有的边。也可以选择1->3->4和2(因为2作为初始站,不需要由1转移过来)。对于一条连续的路径vi1->vi2->…vik由于这条路径上的任务实际上是由一辆taxi来完成的,可以吧这条路径退化成两个点vi1->vik。有了这两步建图的步骤以后,问题的求解就可以变为找出顶点集的一个最小子集,使这个顶点子集覆盖所有的边(每条边都至少和一个顶点集的顶点相连)。这个问题就是图的最小点覆盖。再看这张图,还有一些性质能够让我们更好地求出最小点覆盖。这个图是一个有向无环图,没有自环,就可以拆点,把原先建的图变成一张二分图。
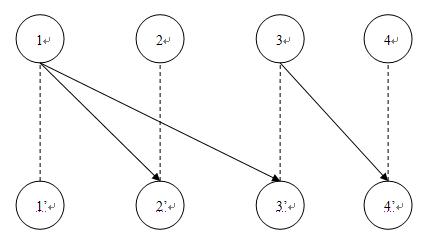
可以再图中看出,上面举出的一条路径1->3->4对应了这个二分图中的路径1->3’->3->4’,在这个二分图中就需要求一个最大独立子集(这里的4点就是一条路径的终点,没一条路径即对应有一个终点!)。二分图的最大独立数是总点数与最大匹配数的差值。接下来建图,拆点,求二分图最大匹配就能解决这道题目了。


1 #include <stdio.h>
#include <stdio.h>
2 #include <string.h>
#include <string.h>
3 #include <algorithm>
#include <algorithm>
4 using namespace std;
using namespace std;
5
6 struct Point
struct Point
7

 {
{
8 int x,y;
int x,y;
9 };
};
10 struct P
struct P
11

 {
{
12 int s,e;
int s,e;
13 struct Point s1,s2;
struct Point s1,s2;
14 }p[505];
}p[505];
15 int n,g[505][505],match[505];
int n,g[505][505],match[505];
16 bool chk[505];
bool chk[505];
17
18
 /**//*int abs(int a)
/**//*int abs(int a)
19 {
{
20 return a < 0 ? -a : a;
return a < 0 ? -a : a;
21 }*/
}*/
22 bool operator <(const P a, const P b)
bool operator <(const P a, const P b)
23

 {
{
24 return a.s < b.s;
return a.s < b.s;
25 }
}
26 int dfs(int v)
int dfs(int v)
27

 {
{
28 int i,t;
int i,t;
29 for(i=0; i<n; i++)
for(i=0; i<n; i++)
30

 {
{
31 if(!chk[i] && g[v][i])
if(!chk[i] && g[v][i])
32

 {
{
33 t=match[i];
t=match[i];
34 chk[i]=true;
chk[i]=true;
35 match[i]=v;
match[i]=v;
36 if(t == -1 || dfs(t)) return 1;
if(t == -1 || dfs(t)) return 1;
37 match[i]=t;
match[i]=t;
38 }
}
39 }
}
40 return 0;
return 0;
41 }
}
42 int Twomatch()
int Twomatch()
43

 {
{
44 int i,ans=0;
int i,ans=0;
45 memset(match,-1,sizeof(match));
memset(match,-1,sizeof(match));
46 for(i=0; i<n; i++)
for(i=0; i<n; i++)
47

 {
{
48 memset(chk,false,sizeof(chk));
memset(chk,false,sizeof(chk));
49 if(dfs(i)) ans++;
if(dfs(i)) ans++;
50 }
}
51 return ans;
return ans;
52 }
}
53
54 int main()
int main()
55

 {
{
56 int i,cases,a,b,j,cnt;
int i,cases,a,b,j,cnt;
57 char temp[10];
char temp[10];
58 //freopen("in.txt","r",stdin);
//freopen("in.txt","r",stdin);
59 scanf("%d",&cases);
scanf("%d",&cases);
60 while(cases--)
while(cases--)
61

 {
{
62 scanf("%d",&n);
scanf("%d",&n);
63
64 for(i=0; i<n; i++)
for(i=0; i<n; i++)
65

 {
{
66 scanf("%s",temp);
scanf("%s",temp);
67 scanf("%d%d%d%d",&p[i].s1.x,&p[i].s1.y,&p[i].s2.x,&p[i].s2.y);
scanf("%d%d%d%d",&p[i].s1.x,&p[i].s1.y,&p[i].s2.x,&p[i].s2.y);
68 sscanf(temp,"%d%*[:]%d",&a,&b);
sscanf(temp,"%d%*[:]%d",&a,&b);
69 p[i].s=a*60+b;
p[i].s=a*60+b;
70 p[i].e=p[i].s+abs(p[i].s2.x-p[i].s1.x)+abs(p[i].s2.y-p[i].s1.y);
p[i].e=p[i].s+abs(p[i].s2.x-p[i].s1.x)+abs(p[i].s2.y-p[i].s1.y);
71 }
}
72 //sort(p,p+n);
//sort(p,p+n);
73 cnt=0;
cnt=0;
74 memset(g,0,sizeof(g));
memset(g,0,sizeof(g));
75 for(i=0; i<n; i++)
for(i=0; i<n; i++)
76

 {
{
77 for(j=0; j<n; j++)
for(j=0; j<n; j++)
78

 {
{
79 if(p[i].e+abs(p[i].s2.x-p[j].s1.x)+abs(p[i].s2.y-p[j].s1.y) < p[j].s && i != j)
if(p[i].e+abs(p[i].s2.x-p[j].s1.x)+abs(p[i].s2.y-p[j].s1.y) < p[j].s && i != j)
80 g[i][j]=1;
g[i][j]=1;
81 }
}
82 }
}
83 printf("%d\n",n-Twomatch());
printf("%d\n",n-Twomatch());
84 }
}
85 return 0;
return 0;
86 }
}
87
posted on 2008-09-15 19:46
飞飞 阅读(1843)
评论(0) 编辑 收藏 引用 所属分类:
ACM/ICPC