Description
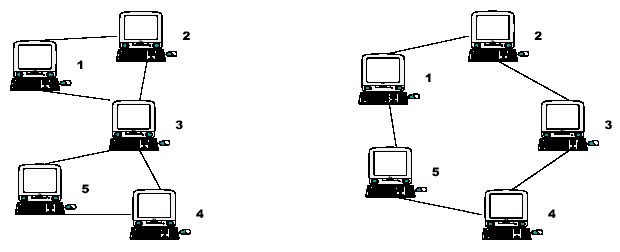
Consider the two networks shown below. Assuming that data moves around these networks only between directly connected nodes on a peer-to-peer basis, a failure of a single node, 3, in the network on the left would prevent some of the still available nodes from communicating with each other. Nodes 1 and 2 could still communicate with each other as could nodes 4 and 5, but communication between any other pairs of nodes would no longer be possible.
Node 3 is therefore a Single Point of Failure (SPF) for this network. Strictly, an SPF will be defined as any node that, if unavailable, would prevent at least one pair of available nodes from being able to communicate on what was previously a fully connected network. Note that the network on the right has no such node; there is no SPF in the network. At least two machines must fail before there are any pairs of available nodes which cannot communicate.
Input
The input will contain the description of several networks. A network description will consist of pairs of integers, one pair per line, that identify connected nodes. Ordering of the pairs is irrelevant; 1 2 and 2 1 specify the same connection. All node numbers will range from 1 to 1000. A line containing a single zero ends the list of connected nodes. An empty network description flags the end of the input. Blank lines in the input file should be ignored.
Output
For each network in the input, you will output its number in the file, followed by a list of any SPF nodes that exist.
The first network in the file should be identified as "Network #1", the second as "Network #2", etc. For each SPF node, output a line, formatted as shown in the examples below, that identifies the node and the number of fully connected subnets that remain when that node fails. If the network has no SPF nodes, simply output the text "No SPF nodes" instead of a list of SPF nodes.
Sample Input
1 2
5 4
3 1
3 2
3 4
3 5
0
1 2
2 3
3 4
4 5
5 1
0
1 2
2 3
3 4
4 6
6 3
2 5
5 1
0
0
Sample Output
Network #1
SPF node 3 leaves 2 subnets
Network #2
No SPF nodes
Network #3
SPF node 2 leaves 2 subnets
SPF node 3 leaves 2 subnets
Source
图论,又是一道割点的题,并且还要求出图中所有的割点分别能将图分割成几个不同的块。可以将某个割点的访问标记设置为1,然后对图进行dfs,方法类似求图中有几个连通的区域。
 #include <iostream>
#include <iostream>
 #include <vector>
#include <vector>
 using namespace std;
using namespace std;

 const int MAXN = 1010;
const int MAXN = 1010;
 bool flag,cut[MAXN],visit[MAXN];
bool flag,cut[MAXN],visit[MAXN];
 vector< vector<int> > adj;
vector< vector<int> > adj;
 int mark[MAXN],deep[MAXN],ancestor[MAXN];
int mark[MAXN],deep[MAXN],ancestor[MAXN];


 void dfs(int u,int father,int depth)
void dfs(int u,int father,int depth) {
{
 int i,v,son=0,len=adj[u].size();
int i,v,son=0,len=adj[u].size();
 mark[u]=1,deep[u]=ancestor[u]=depth;
mark[u]=1,deep[u]=ancestor[u]=depth;

 for(i=0;i<len;i++)
for(i=0;i<len;i++) {
{
 v=adj[u][i];
v=adj[u][i];
 if(v!=father && mark[v]==1)
if(v!=father && mark[v]==1)
 ancestor[u]=min(ancestor[u],deep[v]);
ancestor[u]=min(ancestor[u],deep[v]);

 if(mark[v]==0)
if(mark[v]==0) {
{
 dfs(v,u,depth+1);
dfs(v,u,depth+1);
 son=son+1;
son=son+1;
 ancestor[u]=min(ancestor[u],ancestor[v]);
ancestor[u]=min(ancestor[u],ancestor[v]);
 if((father==-1 && son>1) || (father!=-1 && ancestor[v]>=deep[u]))
if((father==-1 && son>1) || (father!=-1 && ancestor[v]>=deep[u]))
 cut[u]=true;
cut[u]=true;
 }
}
 }
}
 mark[u]=2;
mark[u]=2;
 }
}

 void partition(int u)
void partition(int u) {
{
 visit[u]=true;
visit[u]=true;
 int i,len=adj[u].size();
int i,len=adj[u].size();
 for(i=0;i<len;i++)
for(i=0;i<len;i++)
 if(!visit[adj[u][i]])
if(!visit[adj[u][i]])
 partition(adj[u][i]);
partition(adj[u][i]);
 }
}

 int main()
int main() {
{
 int i,j,x,y,n,cnt,ca=1;
int i,j,x,y,n,cnt,ca=1;

 while(scanf("%d",&x),x)
while(scanf("%d",&x),x) {
{
 scanf("%d",&y);
scanf("%d",&y);
 adj.assign(MAXN,vector<int>());
adj.assign(MAXN,vector<int>());
 n=max(x,y);
n=max(x,y);
 adj[x-1].push_back(y-1),adj[y-1].push_back(x-1);
adj[x-1].push_back(y-1),adj[y-1].push_back(x-1);

 while(scanf("%d",&x))
while(scanf("%d",&x)) {
{
 if(x==0) break;
if(x==0) break;
 scanf("%d",&y);
scanf("%d",&y);
 n=max(x,y);
n=max(x,y);
 adj[x-1].push_back(y-1),adj[y-1].push_back(x-1);
adj[x-1].push_back(y-1),adj[y-1].push_back(x-1);
 }
}
 memset(cut,false,sizeof(cut));
memset(cut,false,sizeof(cut));
 memset(mark,0,sizeof(mark));
memset(mark,0,sizeof(mark));
 for(i=0;i<n;i++)
for(i=0;i<n;i++)
 if(mark[i]==0)
if(mark[i]==0)
 dfs(0,-1,0);
dfs(0,-1,0);
 printf("Network #%d\n",ca++);
printf("Network #%d\n",ca++);
 for(flag=false,i=0;i<n;i++)
for(flag=false,i=0;i<n;i++)

 if(cut[i])
if(cut[i]) {
{
 flag=true;
flag=true;
 memset(visit,false,sizeof(visit));
memset(visit,false,sizeof(visit));
 for(visit[i]=true,cnt=j=0;j<n;j++)
for(visit[i]=true,cnt=j=0;j<n;j++)
 if(!visit[j])
if(!visit[j])
 partition(j),cnt++;
partition(j),cnt++;
 printf(" SPF node %d leaves %d subnets\n",i+1,cnt);
printf(" SPF node %d leaves %d subnets\n",i+1,cnt);
 }
}
 if(!flag)
if(!flag)
 printf(" No SPF nodes\n");
printf(" No SPF nodes\n");
 printf("\n");
printf("\n");
 }
}
 return 0;
return 0;
 }
}