以前从没有对O(log N)和O(N)的区别有所正确认识,今日总算知道了。它们的唯一区别就是,N是一亿的时候,log(N)就是不到26,N还是一亿。
http://acm.pku.edu.cn/JudgeOnline/problem?id=3070
PKU的这道题虽然容易,但的确很有意思。我也是第一次用快速幂取模,一用,果然不同凡响。
快速幂取模,其实就是秦九韶算法 取指数。
把n化成二进制形式后,得到一个多项式,写成秦九韶形式,多项式的加就是乘,乘则为指数运算(指数为2)。由于N的二进制位个数为log(n),这样把O(N)的问题化为O(log N)。
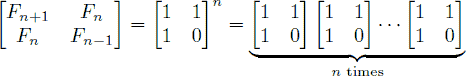 .
.
//PKU 3070 ,calculate Fibonacci
 #include <iostream>
#include <iostream>
 #include<stack>
#include<stack>
 int FPM(int);//fast-power-modulus function declare
int FPM(int);//fast-power-modulus function declare
 using namespace std;
using namespace std;
 const int Mod=10000;
const int Mod=10000;
 int main(int argc, char *argv[])
int main(int argc, char *argv[])


 {
{
 int n=0;
int n=0;
 while(scanf("%d",&n))
while(scanf("%d",&n))


 {
{
 if(n==-1)
if(n==-1)
 break;
break;
 printf("%d\n",FPM(n));
printf("%d\n",FPM(n));
 }
}

 return 0;
return 0;
 }
}
 int FPM(int n)//fast-power-modulus function
int FPM(int n)//fast-power-modulus function


 {
{

 int matr[4]=
int matr[4]= {1,0,0,1};//initialize matrix
{1,0,0,1};//initialize matrix
 stack<bool>dec;//stack to store binary digit
stack<bool>dec;//stack to store binary digit
 while(n)//resolve n to binary digit
while(n)//resolve n to binary digit


 {
{
 dec.push(1&n);//get the last binary digit
dec.push(1&n);//get the last binary digit
 n>>=1;
n>>=1;
 }
}
 while(!dec.empty())
while(!dec.empty())


 {
{
 //matrix square
//matrix square
 matr[1]=((matr[0]+matr[3])*matr[1])%Mod;
matr[1]=((matr[0]+matr[3])*matr[1])%Mod;
 matr[0]=(matr[0]*matr[0]+matr[2]*matr[2])%Mod;
matr[0]=(matr[0]*matr[0]+matr[2]*matr[2])%Mod;
 matr[3]=(matr[3]*matr[3]+matr[2]*matr[2])%Mod;
matr[3]=(matr[3]*matr[3]+matr[2]*matr[2])%Mod;
 matr[2]=matr[1];
matr[2]=matr[1];
 //matrix multiply,
//matrix multiply,
 if(dec.top())
if(dec.top())


 {
{
 matr[0]=(matr[0]+matr[1])%Mod;
matr[0]=(matr[0]+matr[1])%Mod;
 matr[1]=(matr[1]+matr[3])%Mod;
matr[1]=(matr[1]+matr[3])%Mod;
 matr[3]=matr[2];
matr[3]=matr[2];
 matr[2]=matr[1];
matr[2]=matr[1];
 }
}
 dec.pop();
dec.pop();
 }
}
 return matr[1];//matr[1] is the result F[N]
return matr[1];//matr[1] is the result F[N]

 }
}
